Significant figures (simple rule application problems)

Topic outline

• general

  Collapse all Expand all
  • 

    Japanese URL

• Application problems of calculations considering significant figures
  • 
    

    Applied questions:

    　Calculate the content of carbon components contained in particles in seawater (%: carbon content (g)/particle dry weight (g)) and carbon component concentration in seawater (mol (C)/L) in the following measurements with significant figures in mind.
    

     

    　We weighed 1.00L (3 significant figures) of seawater in a volumetric flask, filtered the water through a glass fiber filter, and measured the dry weight of what remained on the filter paper (residue). Next, the filter was burned at high temperature using a platinum catalyst in an oxygen atmosphere, converting all the carbon components into carbon dioxide. The carbon dioxide concentration is measured and converted to the amount of carbon contained in the particulate matter on the filter.

    
    

    

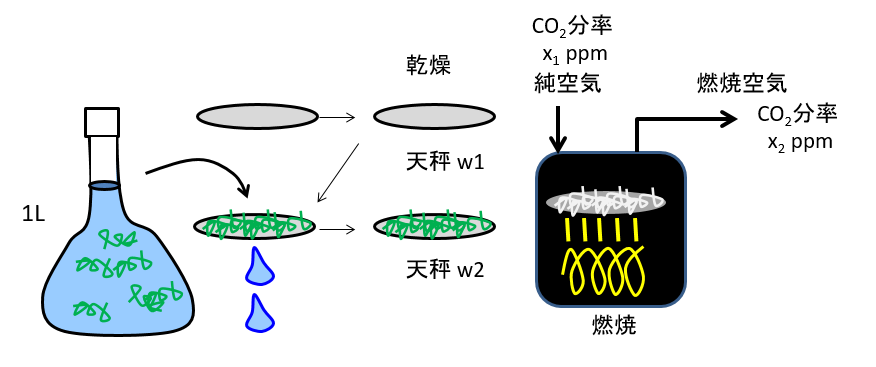
    乾燥Drying　天秤balance　燃焼Combustion　分率Fraction　純空気pure air　燃焼空気combustion air
    

     

    
    

    Dry weight of glass fiber filter (before use)              ：w₁ = 1.0014 g

    Filter dry weight after filtering and drying seawater   ：w₂ = 1.0021 g

                                   (The number of significant figures for w1 and w2 is 6 digits)

     

    Burn the filter at high temperature to generate CO₂ and measure the CO₂ concentration in the combustion air

     

    CO₂ mole fraction in combustion air (before combustion)  ：x₁ = 1 ppm

                                             (1 significant digit)

    CO₂ mole fraction in combustion air (after combustion) ：x₂ = 21040 ppm

                                            (5 significant digits)

    Number of moles of combustion air　                 ：y = 0.013 mol

                                        (2 significant digits)

     

    (Supplement: The number of moles of air (combustion air) sent to the combustion cell can be adjusted with a mass flow controller)

     

    formula

    Dry weight of particles on filter (g)      ：A = w₂ – w₁

    Difference in CO₂ mole fraction in combustion air     ：B = x₂ – x₁  (ppm)

    Number of moles of CO₂ burned                ：C = y×(x₂ – x₁)×10^-6

    Weight of CO₂ burned (g)           ：D = y×(x₂ – x₁)×10^-6/FW_CO2,

                               FW_CO2 is the formula quantity of CO2 (44.01) and is a constant.

    Carbon content (%) in dry particles      ：E = D/A×10² (%)

    Carbon concentration of seawater particles (mol(C)/L)     ：F = C/1.00
    

     
    

    Calculate E and F paying attention to significant figures.

     

    A = 1.0021 – 1.0014

    B = 21040 – 1

    C = 0.013×B×10^-6

    D = 0.013×B×10^-6/44.01

    E = 0.013×B×10^-6/44.01/A ×10² (%)

    F = 0.013×B×10^-6/1.00

     

    First, calculate addition and subtraction

           A = 1.0021 – 1.0014         = 0.0007 (g)

                                      (1 significant digit)

           B = 210400 – 1              = 21039  (ppm)

                                       (5 significant digits)

    (The minimum significant digits of the values used for A and B are the 4th and 1st decimal places, respectively, which are the same. You can just calculate them as is.)

     

             E = 0.013×21039×10^-6/44.01/0.0007×10²  = (0.887807965....)

    Number of significant figures：2,   5,             1 digit(s)

                        *Significant figures are not considered for constant 44.01.

    Smallest number of significant figures: 1 digit

    (Round off the number 0.88780.., which is one digit lower than the lowest significant figure (8).)

     

    Carbon content in dry particles(E)：0.9 (%)　（Number of significant figures: 1 digit）

     

             F = 0.013×21039×10^-6/1.00 = (273.057×10^-6) = 0.000273057)

    Number of significant digits: 2 digits, 5 digits, 3 digits

    2 digits with the smallest number of significant digits

     

    Carbon concentration in seawater particles(F)：2.7×10^-4 (mol(C)/L)　(2 significant digits)

                         (or 0.27 (mmol(C)/L)

    
    

    /////////////////////////////////////////////////////////////////

  • Summary of this applied problem

     

    given number

    Filter dry weight (before use)：w₁ = 1.0014 g        (5 significant digits)

    Filter dry weight (after use)：w₂ = 1.0021 g        (5 significant digits)

    CO2 mole fraction (before combustion)      ：x₁ = 1 ppm         (1 significant digit)

    CO2 mole fraction (after combustion)       ：x₂ = 21040 ppm    (5 significant digits)

    Number of moles of combustion air　          ：y = 0.013 mol     (2 significant digits)

     

    Intermediate calculation

    A = 1.0021 – 1.0014  = 0.0007 (g)                (Number of significant figures: 1 digit)

    B = 210400 – 1       = 21039  (ppm)              (Number of significant figures: 5 digits)

     

    Calculation result

    carbon content(E)：0.9 (%)                             （Number of significant figures: 1 digit）

    carbon concentration(F)：2.7×10^-4 (mol(C)/L)                 （2 significant digits）

     

    The reason why the number of significant figures for carbon content (E) is one digit is due to the number of significant figures (one digit) in intermediate calculation A. Although electronic balances measured weight with six-digit accuracy, the difference was only one digit.

     

    　The reason why the number of significant figures for carbon concentration (F) is 2 digits is because the number of significant figures (2 digits) for the number of moles of combustion air in the given value.

     

    　In this way, by tracking the causes of errors in numerical analysis results, it becomes clear where improvements in analysis operations can lead to improved accuracy. In this example, we found that using a more accurate electronic balance and increasing the accuracy of the number of moles of combustion air (mass flow controller) lead to improved accuracy.