Electrochemistry (Daniel battery)

Topic outline

• General

  Collapse all Expand all
  • 

    Japanese URL

• Standard problems in electrochemistry (Daniel battery)
  • 　Two water tanks each have zinc (Zn) and copper (Cu) electrodes, and 2 mol each of Zn²⁺, Cu²⁺, and SO₄^2- ions are dissolved in the water.

    　(SO42- is present because ZnSO₄ and CuSO₄ are dissolved)
    

    
    

    Example 1-1)

    　Find the standard electrode potential for the half reaction of each electrode.

    answer

    
    

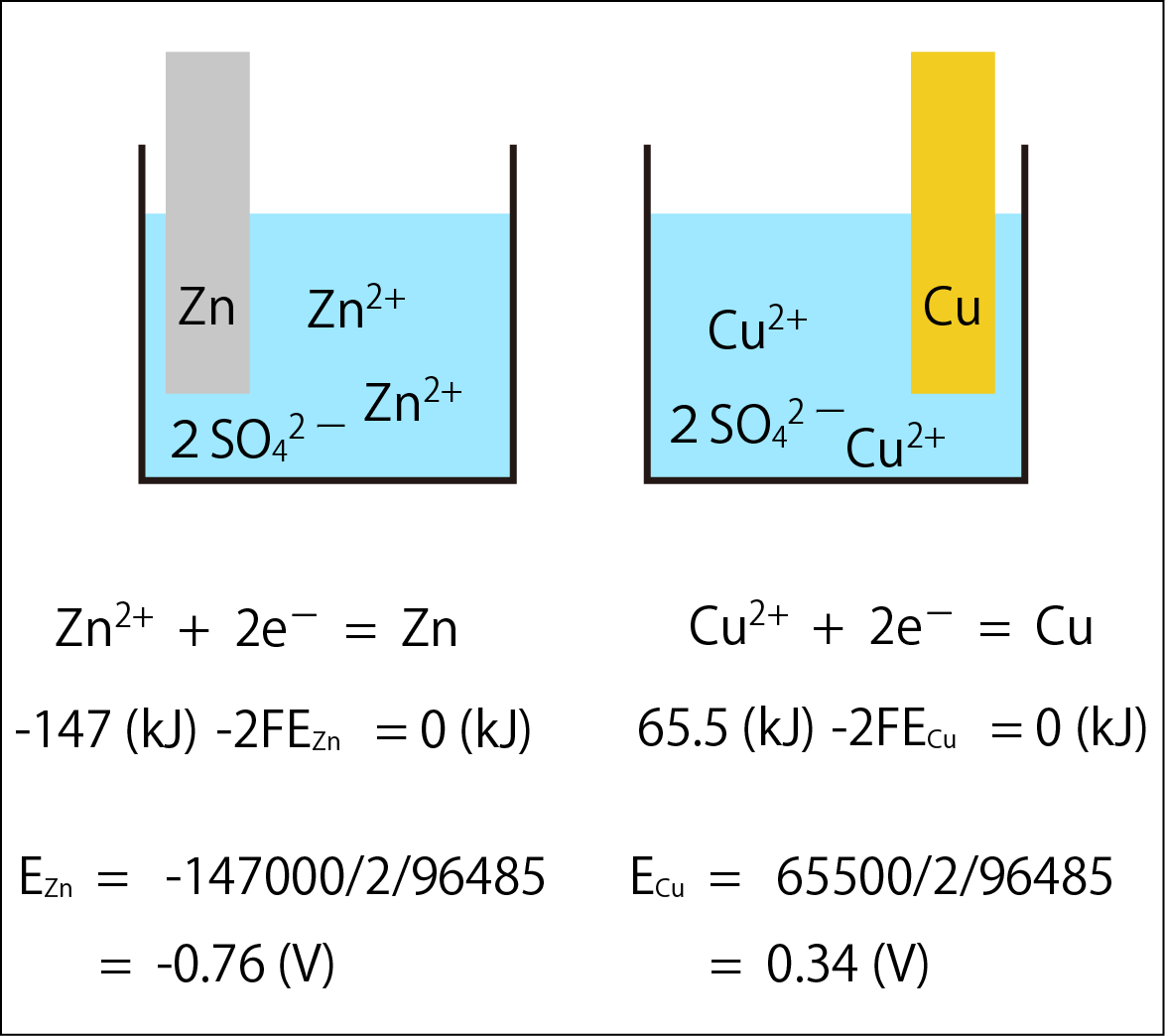
    　The diagram above shows the half-reaction in which copper (Cu) and zinc (Zn) release electrons, the standard Gibbs energy of formation for each substance, and the energy of the electrons. The standard electrode potentials resulting from these half-reactions are calculated as E_Zn = -0.76 (V) and E_Cu = 0.34 (V).

    
    

    　If these electrodes were connected to form a circuit, electrons might flow from the zinc, which has a lower potential, to the copper, which has a higher potential (see the diagram below).
    

    
    

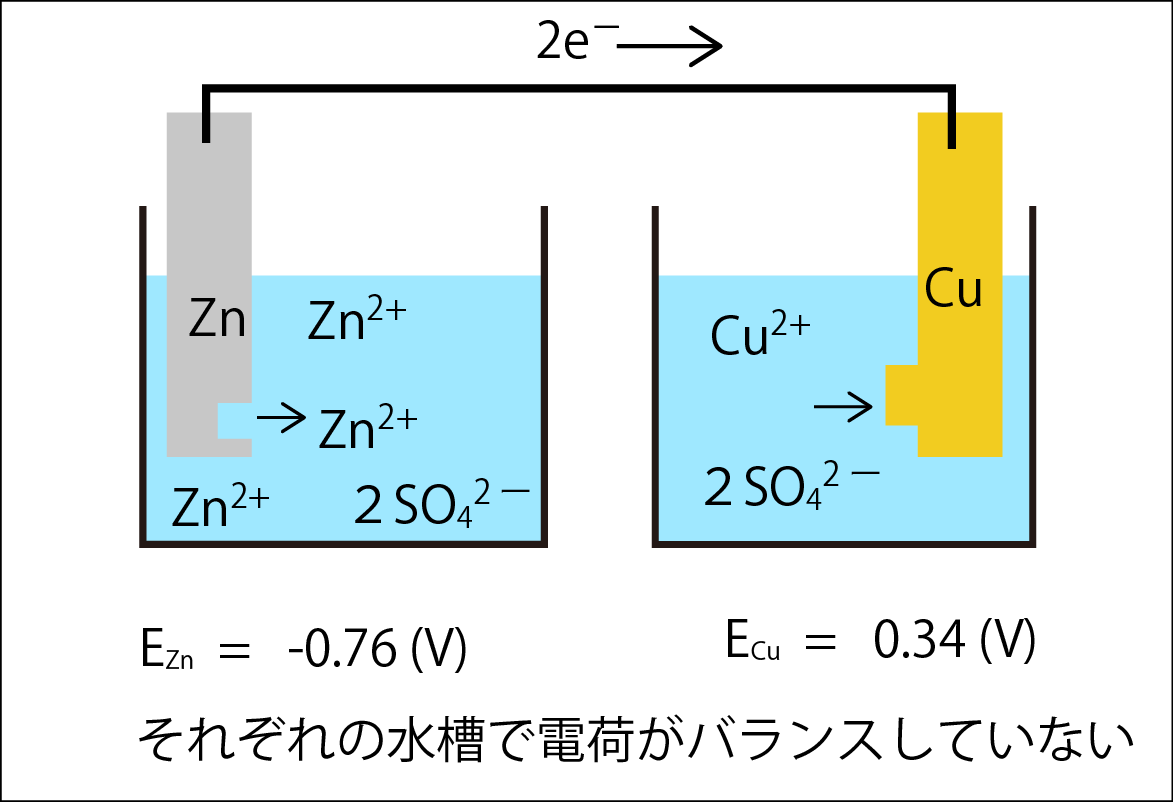
    それぞれの水槽で電荷がバランスしていないCharges are not balanced in each tank　
    

    Unfortunately, this is not a circuit. If this continues, there will be an excess of cations in the zinc tank and an excess of anions in the copper tank, disrupting the charge balance and making it impossible for the reaction to proceed.

     

    　Therefore, to create a circuit, we installed a salt bridge to supply ions to both tanks.

    
    

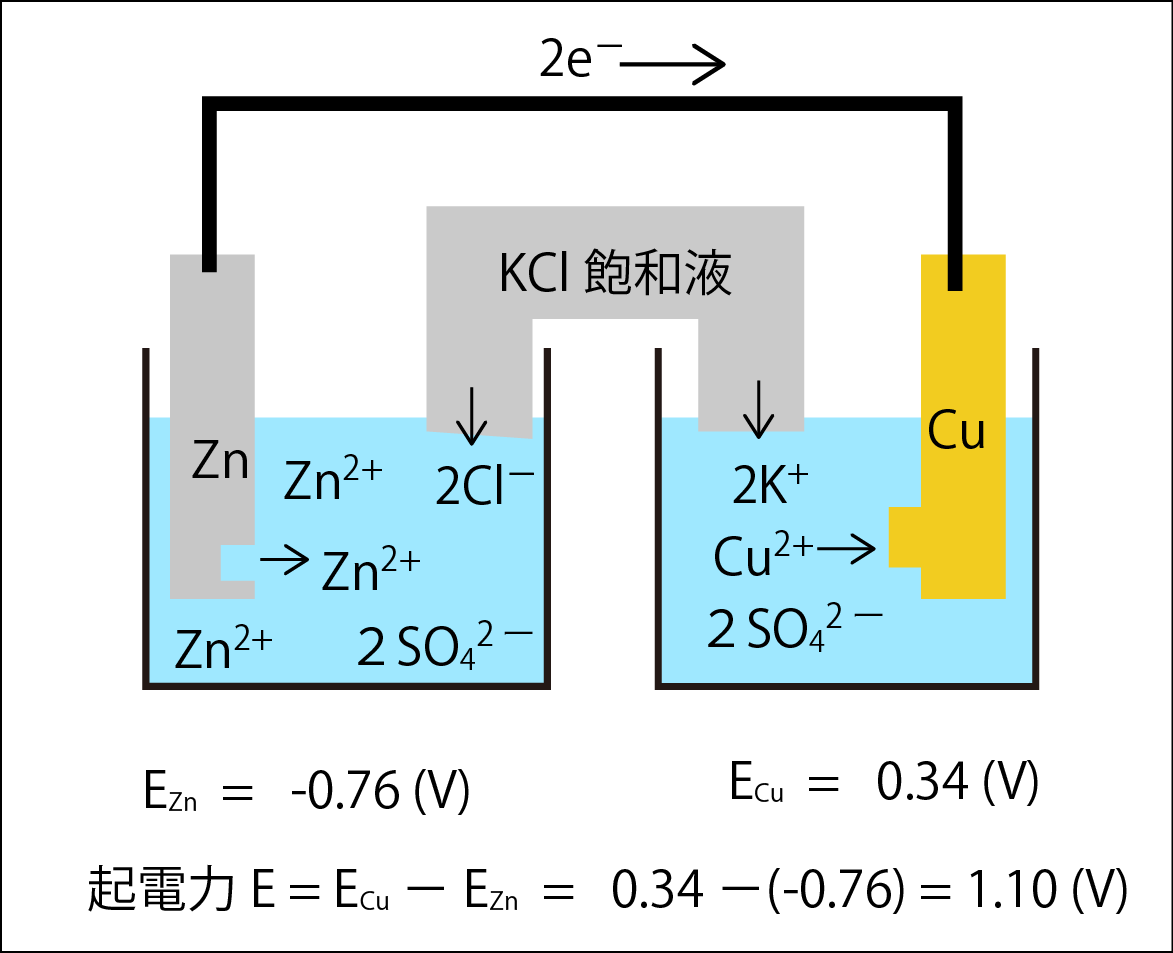
    飽和液saturated liquid　起電力Start electricity
    

    　The salt bridge contains a KCl saturated solution, electrically connecting both tanks and supplying K⁺ and Cl^－to balance the charges. At this time, we ensure that Zn²⁺ and Cu²⁺ do not mix between both tanks. At this time, the difference in standard electrode potential between both water tanks is 1.10 (V).

    
    

    Example 2)　Let us assume that the initial concentration of copper and zinc ions when making the circuit is [Cu²⁺] = [Zn²⁺] = 0.1 (mol/L). As this reaction progressed, [Cu²⁺] = 0.05 (mol/L) and [Zn²⁺] = 0.15 (mol/L). Calculate how the electromotive force changes using Nernst's equation. Similarly, find the electromotive force when [Cu²⁺] = 0.001 (mol/L) and [Zn²⁺] = 0.199 (mol/L).
    answer
    

    
    Substitute unknown [Zn²⁺] and [Cu²⁺] into Nernst's equation. The standard electrode potential is the value determined in Example 1.

    E_Zn = －0.76 － RT/(2F)・Ln([Zn]／[Zn²⁺])

    E_Cu =   0.34 － RT/(2F)・Ln([Cu]／[Cu²⁺])

    Since the activity of a solid is 1,[Zn]=[Cu]=1

    The potential difference (electromotive force) E between the two half reactions is

    E = E_Cu – E_Zn = 0.34 – (-0.76) －RT/(2F)・Ln[Zn²⁺]/[Cu²⁺]

    The electromotive force 1.10 (V) found earlier is when [Zn²⁺]/[Cu²⁺]=1.

    At the initial stage of making the circuit, set [Cu²⁺] = [Zn²⁺] = 0.1. When this reaction progresses and [Cu²⁺] = 0.05 and [Zn²⁺] = 0.15, the electromotive force E becomes

     

    E = 1.10 －RT/(2F)・Ln(0.15/0.05) = 1.09 (V)

    。As the battery reaction progresses further and becomes [Cu²⁺] = 0.01 and [Zn²⁺] = 0.19, E = 1.10 - RT/(2F)・Ln(0.19/0.01) = 1.062 (V), [Cu²⁺] = 0.001 and [Zn²⁺ ] = 0.199, E = 1.032 (V), [Cu²⁺] = 0.000001 and [Zn²⁺] = 0.199999, E = 0.94 (V). A value close to the initial electromotive force (1.10 V) is maintained until just before the battery level reaches zero.

     

    
    

    
    

    In the next course, you will learn the basics of physical chemistry. In the chemical equilibrium equation and Nernst's equation, -Ln (concentration product of formed form/concentration product of original form) is derived.